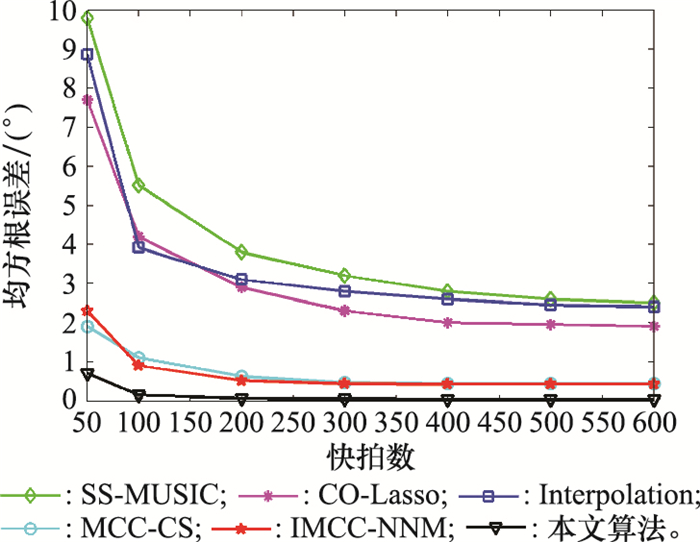
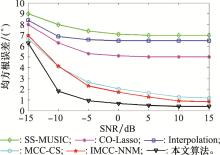
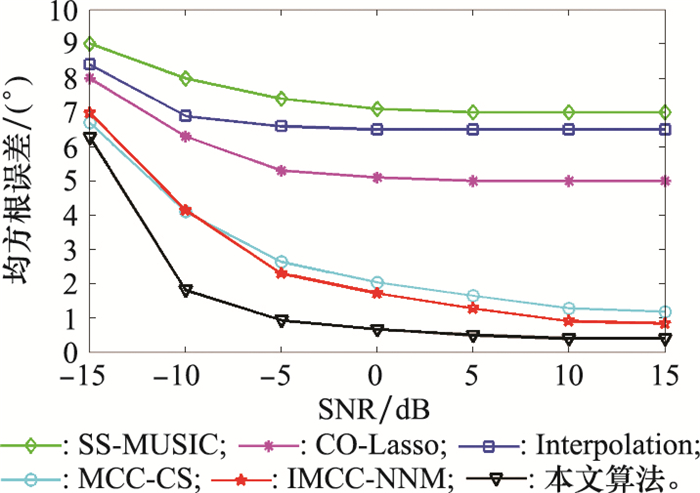
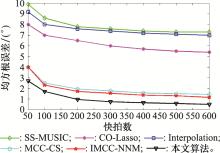
Systems Engineering and Electronics ›› 2021, Vol. 43 ›› Issue (12): 3488-3494.doi: 10.12305/j.issn.1001-506X.2021.12.09
• Sensors and Signal Processing • Previous Articles Next Articles
DOA estimation method for coprime array under gain and phase error
Bing SUN*, Huailin RUAN, Chenxi WU, Shilong WU, Hua ZHONG
- College of Electronic Engineering, National University of Defense Technology, Hefei 230037, China
-
Received:2020-12-25Online:2021-11-24Published:2021-11-30 -
Contact:Bing SUN
CLC Number:
Cite this article
Bing SUN, Huailin RUAN, Chenxi WU, Shilong WU, Hua ZHONG. DOA estimation method for coprime array under gain and phase error[J]. Systems Engineering and Electronics, 2021, 43(12): 3488-3494.
share this article
| 1 |
LIU C L , VAIDYANATHAN P P . Remarks on the spatial smoothing step in coarray MUSIC[J]. IEEE Signal Processing Letters, 2015, 22 (9): 1438- 1442.
doi: 10.1109/LSP.2015.2409153 |
| 2 |
SHI J P , HU G P , ZHANG X F , et al. Sparsity-based DOA estimation of coherent and uncorrelated targets with flexible MIMO radar[J]. IEEE Trans.on Vehicular Technology, 2019, 68 (6): 5835- 5848.
doi: 10.1109/TVT.2019.2913437 |
| 3 |
TANG B , LI J . Spectrally constrained MIMO radar waveform design based on mutual information[J]. IEEE Trans.on Signal Processing, 2019, 67 (3): 821- 834.
doi: 10.1109/TSP.2018.2887186 |
| 4 |
VAIDYANATHAN P P , PAL P . Sparse sensing with co-prime samplers and arrays[J]. IEEE Trans.on Signal Processing, 2011, 59 (2): 573- 586.
doi: 10.1109/TSP.2010.2089682 |
| 5 |
SI Q , ZHANG Y D , AMIN M G . Generalized coprime array configurations for direction-of-arrival estimation[J]. IEEE Trans.on Signal Processing, 2015, 63 (6): 1377- 1390.
doi: 10.1109/TSP.2015.2393838 |
| 6 |
WANG X M , WANG X . Hole identification and filling in k-times extended co-prime arrays for highly-efficient DOA estima-tion[J]. IEEE Trans.on Signal Processing, 2019, 67 (10): 2693- 2706.
doi: 10.1109/TSP.2019.2899292 |
| 7 |
MENG Z , ZHOU W D . Direction-of-arrival estimation in coprime array using the ESPRIT-based method[J]. Sensors, 2019, 19 (3): 707.
doi: 10.3390/s19030707 |
| 8 |
LIU W , RAZA A R , SHEN Q . Thinned coprime array for second-order difference co-array generation with reduced mutual coupling[J]. IEEE Trans.on Signal Processing, 2019, 67 (8): 2052- 2065.
doi: 10.1109/TSP.2019.2901380 |
| 9 | PAL P, VAIDYANATHAN P P. Coprime sampling and the MUSIC algorithm[C]//Proc. of the IEEE Digital Signal Processing and Signal Processing Education Meeting, 2011: 289-294. |
| 10 | ZHANG Y D, AMIN M G, HIMED B. Sparsity-based DOA estimation using co-prime arrays[C]//Proc. of the IEEE International Conference on Acoustics, Speech and Signal Processing, 2013: 3967-3971. |
| 11 |
ZHOU C W , GU Y J , FAN X , et al. Direction-of-arrival estimation for coprime array via virtual array interpolation[J]. IEEE Trans.on Signal Processing, 2018, 66 (22): 5956- 5971.
doi: 10.1109/TSP.2018.2872012 |
| 12 | HU B , WU X C , ZHANG X , et al. DOA estimation based on compressed sensing with gain/phase uncertainties[J]. IET Radar, Sonar & Navigation, 2018, 12 (11): 1346- 1352. |
| 13 |
CAMLICA S , YETIK I S , ARIKAN O . Sparsity based off-grid blind sensor calibration[J]. Digital Signal Processing, 2019, 84, 80- 92.
doi: 10.1016/j.dsp.2018.10.005 |
| 14 |
LIU H Q , ZHAO L M , LI Y , et al. A sparse-based approach for DOA estimation and array calibration in uniform linear array[J]. IEEE Sensors Journal, 2016, 16 (15): 6018- 6027.
doi: 10.1109/JSEN.2016.2577712 |
| 15 | WEISS A J , FRIEDLANDER B . Eigenstructure methods for direction finding with sensor gain and phase uncertainties[J]. Circuits Systems & Signal Processing, 1990, 9 (3): 271- 300. |
| 16 |
LIU A F , LIAO G S , ZENG C , et al. An eigenstructure method for estimating doa and sensor gain-phase errors[J]. IEEE Trans.on Signal Processing, 2011, 59 (12): 5944- 5956.
doi: 10.1109/TSP.2011.2165064 |
| 17 |
LIU A F , LIAO G S . An eigenvector based method for estimating DOA and sensor gain-phase errors[J]. Digital Signal Processing, 2018, 79, 116- 124.
doi: 10.1016/j.dsp.2018.04.013 |
| 18 |
WANG B , ZHANG Y D , WANG W . Robust DOA estimation in the presence of miscalibrated sensors[J]. IEEE Signal Processing Letters, 2017, 24 (7): 1073- 1077.
doi: 10.1109/LSP.2017.2708659 |
| 19 |
KOU J X , LI M , JIANG C L . A robust DOA estimator based on compressive sensing for coprime array in the presence of miscalibrated sensors[J]. Sensors, 2019, 19 (16): 3538.
doi: 10.3390/s19163538 |
| 20 |
KOU J X , LI M , JIANG C L . Robust direction-of-arrival estimation for coprime array in the presence of miscalibrated sensors[J]. IEEE Access, 2020, 8, 27152- 27162.
doi: 10.1109/ACCESS.2020.2971842 |
| 21 |
孙兵, 吴晨曦, 阮怀林, 等. 阵元失效条件下的高精度DOA估计方法[J]. 电子学报, 2020, 48 (9): 1688- 1694.
doi: 10.3969/j.issn.0372-2112.2020.09.004 |
|
SUN B , WU C X , RUAN H L , et al. High accuracy DOA estimation method with array sensor failure[J]. Acta Electronica Sinica, 2020, 48 (9): 1688- 1694.
doi: 10.3969/j.issn.0372-2112.2020.09.004 |
|
| 22 |
SUN B , WU C X , RUAN H L . Array diagnosis and DOA estimation for coprime array under sensor failures[J]. Sensors, 2020, 20 (9): 2735.
doi: 10.3390/s20092735 |
| 23 |
WENG Z Y , DJURIĆP M . A search-free DOA estimation algorithm for coprime arrays[J]. Digital Signal Processing, 2014, 24, 27- 33.
doi: 10.1016/j.dsp.2013.10.005 |
| 24 |
SUN F G , GAO B , CHEN L Z , et al. A low-complexity ESPRIT-based DOA estimation method for co-prime linear arrays[J]. Sensors, 2016, 16 (9): 1367.
doi: 10.3390/s16091367 |
| 25 |
YAN F G , LIU S , WANG J , et al. Fast DOA estimation using co-prime array[J]. Electronics Letters, 2018, 54 (7): 409- 410.
doi: 10.1049/el.2017.2491 |
| 26 |
王鼎, 潘苗, 吴瑛. 基于辅助阵元的方位依赖幅相误差最大似然自校正: 针对确定信号模型[J]. 通信学报, 2011, 32 (2): 34- 41.
doi: 10.3969/j.issn.1000-436X.2011.02.005 |
|
WANG D , PAN M , WU Y . Maximum likelihood self-calibration for direction-dependent gain-phase errors with carry-on instrumental sensors: case of deterministic signal model[J]. Journal on Communications, 2011, 32 (2): 34- 41.
doi: 10.3969/j.issn.1000-436X.2011.02.005 |
|
| 27 | 王布宏, 王永良, 陈辉, 等. 方位依赖阵元幅相误差校正的辅助阵元法[J]. 中国科学: 技术科学, 2004, 34 (8): 906- 918. |
| WANG B H , WANG Y L , CHEN H , et al. Calibration for direction-dependent gain-phase errors with carry-on instrumental sensors[J]. Scientia Sinica (Technologica), 2004, 34 (8): 906- 918. | |
| 28 | 孙兵, 阮怀林, 吴晨曦, 等. 基于Toeplitz协方差矩阵重构的互质阵列DOA估计方法[J]. 电子与信息学报, 2019, 41 (8): 1924- 1930. |
| SUN B , RUAN H L , WU C X , et al. Direction of arrival estimation with coprime array based on toeplitz covariance matrix reconstruction[J]. Journal of Electronics & Information Technology, 2019, 41 (8): 1924- 1930. | |
| 29 |
CANDÈS E J , RECHT B . Exact matrix completion via convex optimization[J]. Foundations of Computational Mathematics, 2009, 9, 717- 772.
doi: 10.1007/s10208-009-9045-5 |
| 30 |
CAI J F , CANDÈS E J , SHEN Z W . A singular value thresholding algorithm for matrix completion[J]. SIAM Journal on Optimization, 2010, 20 (4): 1956- 1982.
doi: 10.1137/080738970 |
| [1] | Jialei LIU, Jiazhi MA, Longfei SHI. DOA estimation algorithm based on fourth-order cumulant using virtual beam forming [J]. Systems Engineering and Electronics, 2022, 44(7): 2134-2142. |
| [2] | Zhiyu QU, Meng SUN, Huanyao DAI. Joint estimation algorithm of DOA and polarization information based on conformal array [J]. Systems Engineering and Electronics, 2022, 44(6): 1798-1804. |
| [3] | Juan WEI, Shian YAN, Fangli NING. DOA estimation method for coherent signals based on coprime array virtual array spatial smoothing [J]. Systems Engineering and Electronics, 2022, 44(4): 1069-1077. |
| [4] | Yajie XU, Yong XIAN, Bangjie LI, Leliang REN, Shaopeng LI, Weilin GUO. Method for improving the precision of hypersonic vehicle inertial navigation system based on neural network [J]. Systems Engineering and Electronics, 2022, 44(4): 1301-1309. |
| [5] | Baohua FAN, Le ZUO, Yong TANG, Zehua HU. DOA estimation of multiple time-varying signals with expectation-maximization algorithm [J]. Systems Engineering and Electronics, 2022, 44(2): 420-426. |
| [6] | Tao CHEN, Lin SHI, Mengyu SHEN. Gridless DOA estimation algorithm based on M-FIPM [J]. Systems Engineering and Electronics, 2022, 44(2): 427-433. |
| [7] | Jun ZHANG, Xinyu ZHANG, Weidong JIANG, Yongxiang LIU, Xiang LI. Fast DOA estimation method using generalized approximate message passing [J]. Systems Engineering and Electronics, 2022, 44(10): 2995-3002. |
| [8] | Sunyong WU, Baohong ZOU, Qiutiao XUE, Xiyan SUN, Li WANG. DOA tracking algorithm based on single snapshot spatial smoothing with multi-Bernoulli [J]. Systems Engineering and Electronics, 2021, 43(9): 2430-2438. |
| [9] | Yili HU, Yongbo ZHAO, Sheng CHEN, Chenghu CAO. Blind polarization DOA estimation of parabolic conformal array based on interpolation fitting [J]. Systems Engineering and Electronics, 2021, 43(8): 2037-2044. |
| [10] | Junpeng SHI, Fangqing WEN, Lin AI, Gong ZHANG, Zhenghui GONG. Angle estimation for bistatic MIMO radar with spatially colored noise [J]. Systems Engineering and Electronics, 2021, 43(6): 1477-1485. |
| [11] | Zixin ZHANG, Guoping HU, Hao ZHOU, Chenghong ZHAN. Low elevation angle estimation algorithm for MIMO radar based on sparse reconstruction of cross-covariance [J]. Systems Engineering and Electronics, 2021, 43(5): 1218-1223. |
| [12] | Silei CAO, Weigui ZENG, Huiqi XU. Broadband DOA estimation method based on eigenvector space focusing [J]. Systems Engineering and Electronics, 2021, 43(2): 294-299. |
| [13] | Chao DING, Weiguo DAI, Sen WANG, Yusheng CHENG. Optimal disposition of vector submerged buoy array based on geometrical dilution of precision [J]. Systems Engineering and Electronics, 2021, 43(11): 3107-3117. |
| [14] | Yule ZHANG, Guoping HU, Hao ZHOU, Shijie YUE, Feilong ZHAO. DOA estimation of generalized two-level nested MIMO radar with high degree of freedom and low mutual coupling [J]. Systems Engineering and Electronics, 2021, 43(10): 2819-2827. |
| [15] | Donghua HUANG, Yongsheng ZHAO, Yongjun ZHAO. Target localization algorithm from DOA-TDOA measurements in passive radar with transmitter and receiver position errors [J]. Systems Engineering and Electronics, 2020, 42(9): 1961-1968. |
| Viewed | ||||||
|
Full text |
|
|||||
|
Abstract |
|
|||||